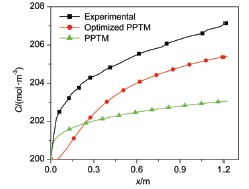
| [1] Wang, X.-L.; Tu, C.-H.; Fang, Y.-Y.; Xiao, Y.; Zhou, B. Membr. Sci. Tech. 2011, 31(3), 127. (王晓琳, 涂丛慧, 方彦彦, 肖燕, 周波, 膜科学与技术, 2011, 31(3), 127.)[2] Hou, L.-A.; Liu, X.-F. Membr. Sci. Tech. 2010, 30(4), 1. (侯立安, 刘晓芳, 膜科学与技术, 2010, 30(4), 1.)[3] Bowen, W. R.; Mukhtar, H. J. Membr. Sci. 1996, 112, 263.[4] Bowen, W. R.; Mohammad, A. W.; Hilal, N. J. Membr. Sci. 1997, 126(1), 91.[5] Déon, S.; Dutournié, P.; Bourseau, P. AICHE J. 2007, 53(8), 1952.[6] Déon, S.; Dutournié, P.; Bourseau, P. Ind. Eng. Chem. Res. 2007, 46(21), 6752.[7] Escoda, A.; Déon, S.; Fievet, P. J. Membr. Sci. 2011, 378(1-2), 214.[8] Deen, W. M. AIChE J. 1987, 33(9), 1409.[9] Gozálvez-Zafrilla, J. M.; Santafé-Moros, A. Desalination 2010, 250(2), 840.[10] Vezzani, D.; Bandini, S. Desalination 2002, 149(1-3), 477.[11] Bird, R. B.; Stewart, W. E.; Lightfoot, E. N. Transport Phenomena, Vol. 2, John Wiley & Sons, New York, 2002, Chapter 5.5.[12] Schaep, J.; Vandecasteele, C.; Mohammad, A. W.; Bowen, W. R. Sep. Sci. Technol. 1999, 34(15), 3009.[13] Fu, S.; Yu, Y.-X.; Gao, G.-H.; Wang, X.-L. Acta Chim. Sinica 2006, 64(22), 2241. (付升, 于养信, 高光华, 王晓琳, 化学学报, 2006, 64(22), 2241.)[14] Xu, Y.-Y.; Xu, Z.-K. Polymeric Membrane Materials, Chemical Industry Press, Beijing, 2005. (徐又一, 徐志康, 高分子膜材料, 化学工业出版社, 北京, 2005.) |